LS Instruments

Introduction

Diffusing Wave Spectroscopy (DWS) is a modern light scattering technique that is mainly utilized to probe the motion of particles in colloidal media.^[1] A microrheological analysis of the particle motion may further provide the medium’s rheological properties, namely the frequency-dependent storage and loss moduli, G’(ω) and G’’(ω), respectively.^[2] DWS can be applied to a rich variety of soft-matter systems, including gelling systems. In particular, DWS is able to accurately characterize their gel point, defined when the normalized intensity correlation function (ICF) no longer decays to zero^[3]. Gelatin solutions (Figure 1a) are dispersions of biopolymers in water that form physical gels below the gelation temperature T_gel (Figure 1b).^[4,5] Furthermore, at the gelation temperature of polymeric gel-forming liquids, G’ ~ G’’ ~ ωⁿ, where 0.19 < n < 0.92.^6] In this application note, we propose to determine T_gel of a food grade gelatin solution using the DWS RheoLab from LS Instruments.

Figure 1. a) liquid and b) gel phases of the gelatin solutions containing 1 wt% of 784 nm diameter polystyrene particles.

Sample preparation

Commercial gelatin was dissolved at 2.5 wt% (weight by weight) in deionized water at T = 50°C. To perform DWS on this transparent mixture, 784 nm diameter tracer polystyrene particles (microParticles GmbH^®) were added at a concentration of 1 wt%. At such a particle concentration, the sample appears white (Figure 1). Subsequently, the solution was introduced into a 5 mm thick glass cuvette at 50°C, which was loaded in the DWS RheoLab. Then, the gelatin solution was progressively cooled from 50°C to 15°C, with temperature steps of 5°C down to 30°C, and steps of 0.5°C from 30°C. At each temperature, a waiting time of 30 min was observed before measuring. The cooling rate was shown not to influence the gel point of gelatin.^[7,8] The transport mean free path l* of the sample was determined using the DWS RheoLab; l* was 290 μm over the temperature range studied.

Results and Discussion

In the liquid phase above T_gel, the ICFs, obtained in transmission, decay to zero, but remain finite in the gel phase below T_gel (Figure 2a). As the sample is further cooled in the gel phase, however, the height H of the plateau of the ICF curves at large values of τis observed to increase (Figure 2). To quantify the latter observation, the normalized ICFs were fitted using the following function: f(τ) = A exp(-τ/τ_C) + H, where A and τ_c are the amplitude and the characteristic time of the decay, respectively. According to Schurtenberger et al.,^[3] the definition of the gel point is when H rises from zero. Therefore, T_gel = 22.5°C.

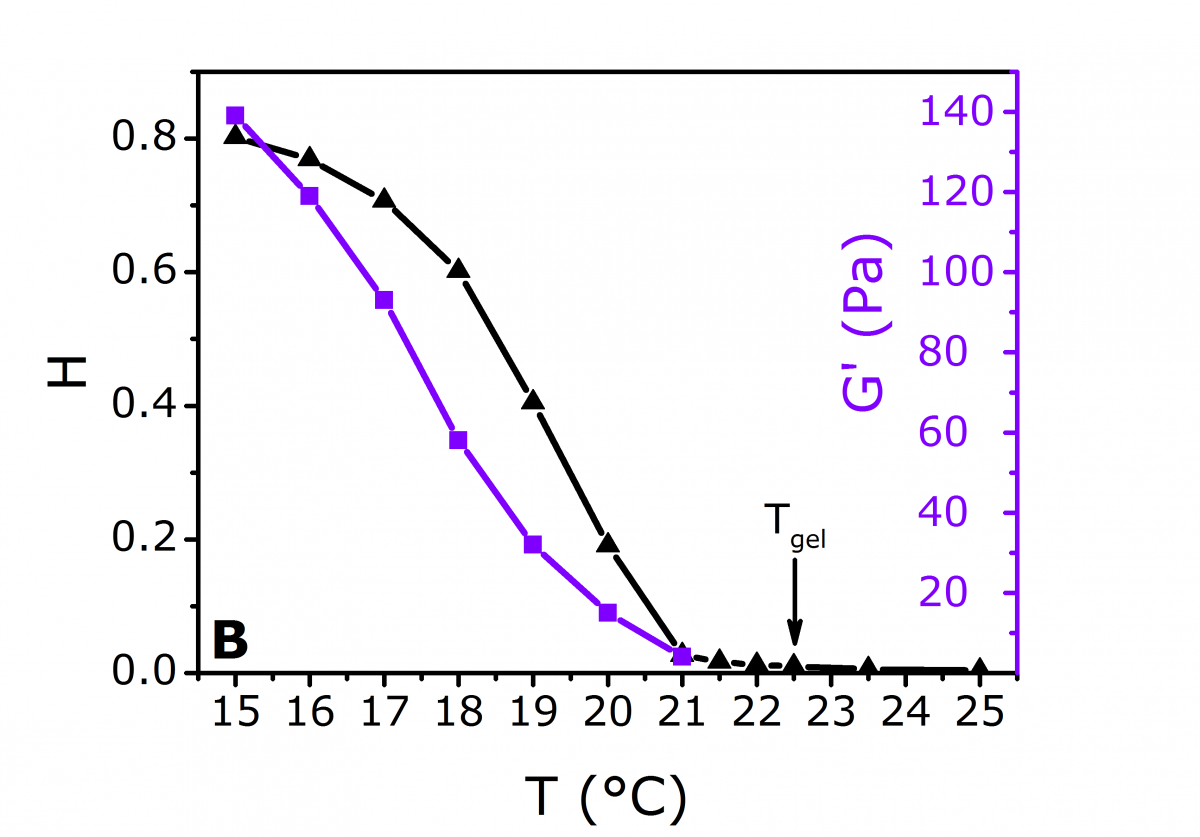Figure 2. a) ICFs measured when cooling the sample (an arrow indicates the direction of the curve change), b) H and G’(ω=100 rad.s^-1) vs. T.

In addition, the increase of H with a further decrease of T to 15°C is a consequence of the progressively restricted particle motion. This is due to the strengthening of the forming gel, as shown in Figure 2b, where H follows the same trend as the elastic modulus G’, taken at ω = 100 rad.s^-1. After automatically extracting the particle mean square displacement (MSD) from the ICFs (Figure 3a), the DWS RheoLab software can accurately compute both G’(ω) and G’’(ω) upon application of the Generalized Stokes-Einstein Relation (GSER) to the particle MSD (Figure 3b).^[2]In the liquid phase, the MSD and G’’ are linear with τ and ω respectively, indicating a purely viscous fluid. In the gel phase, the MSD and G’ saturate at long τ and low ω values respectively. This reflects the elastic behavior of the medium. At T_gel as defined above, MSD ~ τ^0.8, and the relation G’ ~ G’’ ~ ωⁿ with n = 0.8 is verified. Moreover, the magnitude of n is consistent with values previously obtained for similar systems.^[4-5]

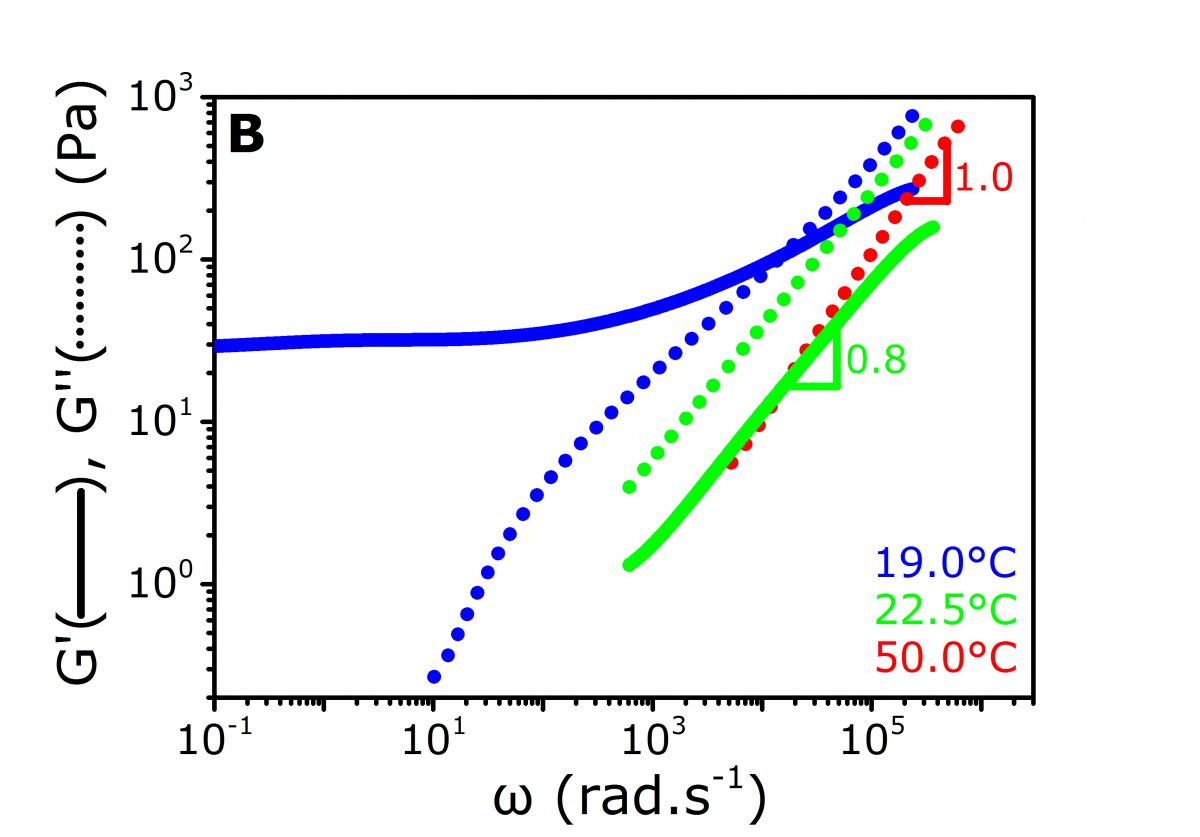Figure 3. a) MSDs, b) Microrheology curves computed by the DWS RheoLab software from the MSDs using the GSER.

Conclusion

Using the DWS RheoLab, we have successfully and unambiguously determined the gelation temperature T_gel of a commercial, food-grade gelatin solution as T_gel= 22.5°C. Aging of gelatin gels could be further studied using the DWS RheoLab since it facilitates time‑dependent measurements.

References

[1] D.A. Weitz, D.J. Pine, Diffusing-Wave Spectroscopy. In Dynamic Light Scattering; Brown, W., Ed.; Oxford University Press: New York, 1993, 652-720.
[2] T.G.Mason, D.A.Weitz, Optical Measurements of Frequency-Dependent Linear Viscoelastic Moduli of Complex Fluids, Phys. Rev. Lett., 1995, 74, 1250-1253.
[3] S. Romer, F. Scheffold, P. Schurtenberger, Sol-Gel Transition of Concentrated Colloidal Suspensions, Phys. Rev. Lett., 2000,85, 4980-4983.
[4] J. Peyrelasse, M. Lamarque, J.P. Habas, N. El Bounia, Rheology of Gelatin Solutions at the Sol-Gel Transition, Phys. Rev. E, 1996, 53, 6126-6133.
[5] T. Matsunaga, M. Shibayama, Gel Point determination of Gelatin Hydrogels by Dynamic Light Scattering and Rheological Measurements, Phys. Rev. E, 2007, 76, 030401 (R).
[6] R.G. Larson, The Structure and Rheology of Complex Fluids. Gubbins K.E., Ed.; Oxford University Press: New York, 1999, 232-260.
[7] H.B. Bohidar, S.S. Jena, Kinetics of sol-gel transition in thermoreversible gelation of gelatin, J. Chem. Phys., 1993, 98, 8970-8977.
[8] The gel point of other colloidal systems could be cooling-rate dependent.