Dilute and Concentrated Microgel Suspensions Studied by 3D Cross-Correlation Techniques
Related Product
NanoLab 3D™
The NanoLab 3D™ is a compact DLS instrument for particle sizing that is based on the groundbreaking and patented Modulated 3D Cross-Correlation technology. It efficiently suppresses multiple light scattering and therefore sample dilution is no longer required for most samples.
Introduction
Gel particles are cross-linked polymeric structures that undergo a volume transition upon change of external stimuli [1], including temperature [2], pH [3], external pressure [4] or radiation [5]. Microgel particles are gels in the colloidal domain, and as a result their properties can be determined using light scattering [6], microscopy [7] and rheology [8]. In addition, due to their smaller size compared to macroscopic gels, they exhibit a fast respond to changes in external stimuli, making them suitable for many technological applications [9].
Microgels are soft particles that are able to deform and shrink at either high packings or under external flows or shear. This remarkable property has a profound influence over their phase behavior [10] and rheological properties [11], emphasizing the differences with hard-sphere-like colloids [12]. A clear example of this distinctive behavior results from considering the maximum packing of hard spheres in a random fashion, which corresponds to a volume fraction of 0.64. If crystallization is allowed, the maximum packing fraction raises to 0.74, which corresponds to a FCC or HCP arrangement. By contrast, when microgel particles are driven to high packing concentrations, they start to deform and, thus, it is possible to keep concentrating the system up to volume fractions of 1. But you can still introduce more particles in the system to hypothetically raise the volume fraction above 1; of course, this corresponds to de-swelling of the particles caused by the steric repulsion with other particles.
To better understand the behavior of microgel particles in these high-concentration regimes, we use light and neutron scattering. From the mean intensity or the correlation of the scattered radiation, one can learn structural and dynamical aspects of the sample probed. The main drawback of the scattering technique comes from the restriction of the theory to simple scattering events. The presence of some multiple scattering in the system can dramatically alter the final result, complicating the interpretation of the data. In the case of neutron scattering, the properties of the solvent can be tuned to change the optical contrast in order to avoid multiple scattering events. In addition, since in many occasions scattering occurs due to neutron-nucleus interactions, even very high concentrations can be probed under simple scattering conditions. For light, however, this is not the case and already scattering from samples with very low concentrations often contains both simple and multiple scattering contributions.
Here we use auto- and cross-correlation schemes to study the dynamics of dilute and concentrated microgel suspensions. In particular, we use the so-called 3D correlation set-up and use the 3D DLS equipment from LS Instruments. As with other cross-correlation techniques the idea is to perform two independent scattering experiments at the same time with the same scattering wave vector, q [13]. In this case, the contributions coming from multiple scattering events do not contribute to the cross-correlation and only affect the value of the background signal, thus allowing the extraction of single scattering.
Experimental results:
One of the experimental systems we use is based on N-Isopropylacrilamide (NIPAM) copolymerized with a 5% concentration of acrylic acid (Acc) and a 2% concentration of cross-linker. This particular combination of monomers allows us to vary the particle size by changing the pH and the temperature.
We determine the size of these PNIPAM-based particles as a function of temperature T, by measuring the intensity correlation function, g2(t), which we convert into the dynamic structure factor, g1(t), using the Siegert relation [6]. Since we use dilute particle concentrations in these experiments, there are no interparticle interactions and [6], where D is the diffusion coefficient of the particle and
,
with l the wavelength of the light in vacuum, n the refractive index of the medium and θ the scattering angle. We observe that g1(t) is indeed an exponential function of time, as shown by the fit in Fig. 1(a), which we use to obtain D, which we further employ to calculate the particle radius a, from the Stokes-Einstein relation,
;
the resultant size-temperature curve is shown in Fig. 1(b).
As expected for any PNIPAM-based system, a temperature increase results in particle de-swelling. The transition temperature is higher than that typically observed for pure PNIPAM microgels, which we attribute to the ionic content provided by Acc; this ionic contribution raises the osmotic pressure demanding a higher temperature in order to induce the particle de-swelling [14]. We emphasize that despite the particle concentration is low in these experiments, there are important contributions from multiple scattering; we thus performed these measurements using cross-correlation to extract the simple scattering from these samples [15]. We also use microgel particles based on vinyl-pyridine (VP). These microgels are ionic in nature, as VP ionizes at low enough pH. We choose this experimental system to elucidate the role played by the elasticity of the individual particle in the macroscopic behavior of the suspension; this requires the knowledge of the single-particle elasticity. To address this, we use dextrans and monitor how the microgel particle de-swells as a function of dextran concentration, which we know corresponds to a certain osmotic pressure [17].
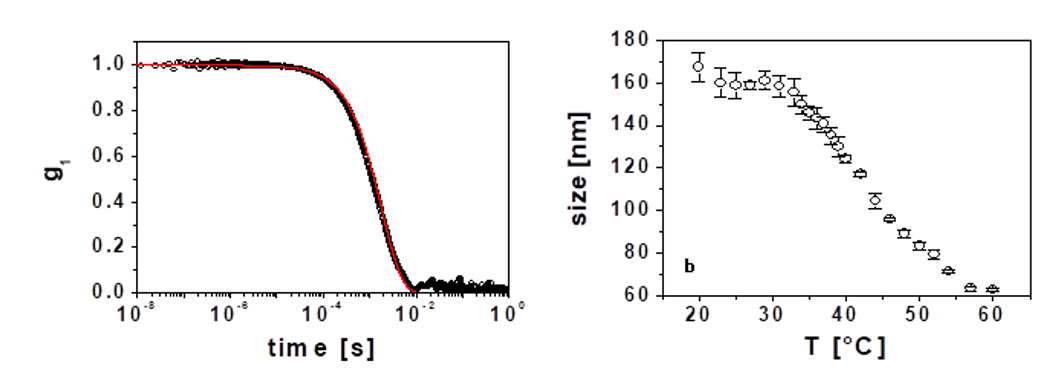
Figure 1. (a) Dynamic structure factor obtained in cross-correlation for a dilute suspension of NIPAM-based particles at T=31°C and pH=5.5. The red line is an exponential fit. (b) Microgel size as a function of temperature.
We first characterize the dextran solution at different concentrations by determining g1(t) using pseudo cross-correlation [16]. For these suspensions, there is no multiple scattering since the microgel particles are highly swollen, implying there is a small refractive index mismatch between the particles and the solvent. We find there are two decays at widely separated time scales, as shown in Fig. 2(a); this is consistent with previous studies on a similar system [18]. As a result, we fit our data to a double exponential of the form:
where Aslow and Afast are the amplitudes of the slow and the fast modes, respectively, and Gslow and Gfast are the corresponding relaxation frequencies. This model correctly describes our data, as shown in Fig. 2(b), where we plot g1(t), as well as the slow and fast modes contributing to g1(t).
We then measure the dynamic structure factor of a microgel suspension in the presence of certain dextran concentration, as shown in Fig. 3(a) for a microgel concentration of 0.02% by weight.
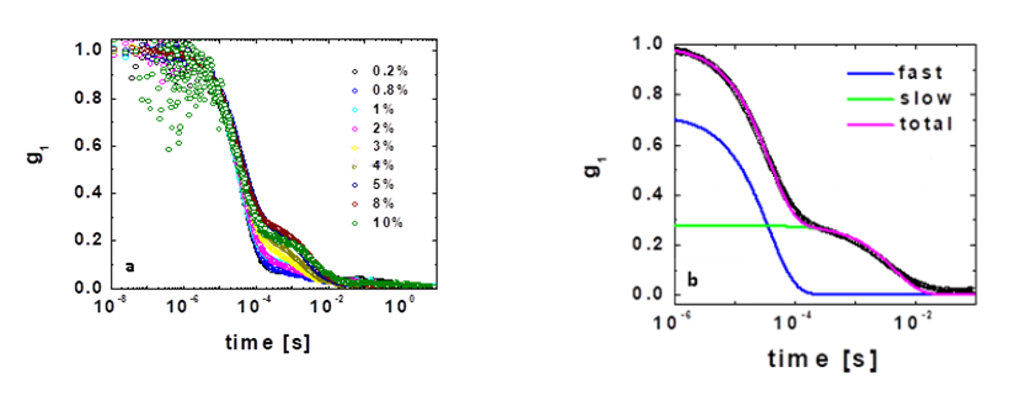
Figure 2. (a) Dynamic structure factor of a dextran solution at different concentrations. (b) Experimental fit using the superposition of two exponential functions, which correspond to the fast and slow decays observed.
In this case, it suffices to perform autocorrelation experiments, as there is also negligible multiple scattering; this, however, restricts the lowest accessible time scales to those above the times associated to detector afterpulsing. The influence of the dextrans to the field correlation function of the microgel suspension is evident.
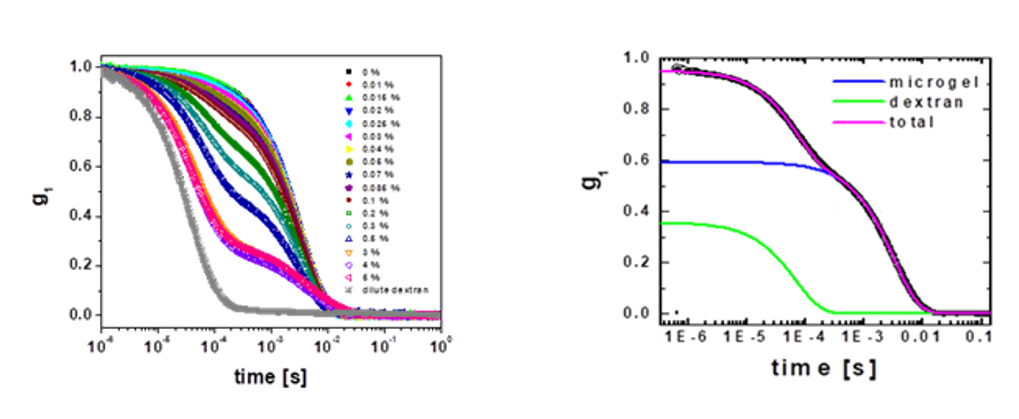
Figure 3. (a) Dynamic structure factor obtained using auto-correlation for VP microgels in the presence of certain dextran concentration. The microgel concentration is 0.02% by weight. (b) Experimental fit of the data based on the superposition of three exponential functions, which correspond to the slow and fast modes of the dextran solution and to the diffusion of the microgel particles. Since the amplitude of the slow mode of the dextran solution is much smaller than that of the microgel particles, this contribution is not shown.
While in the absence of dextrans, g1(t) is characterized by a single exponential decay, at higher dextran concentrations, g1(t) is characterized by two decays. In order to estimate the relaxation frequency associated to the microgel diffusion, we fit g1(t) to a triple exponential form:
where the first two modes correspond to the dextran solution, and the third mode corresponds to the microgel suspension. In the fit, we fix the relaxation frequencies of the dextran at a given concentration, while leaving the amplitudes and the relaxation frequency of the microgel suspensions as free parameters. Our model correctly describes the experimental result, as shown in Fig. 3(b). From the fitted Gmgel, we calculate the diffusion coefficient and thus the particle radius.
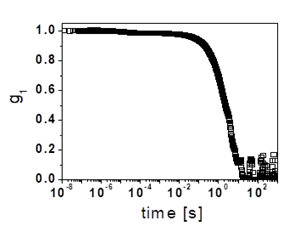
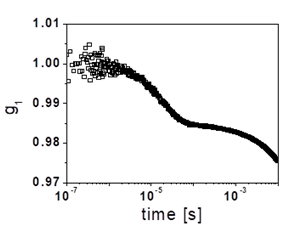
Figure 4. (a) Dynamic structure factor obtained in cross-correlation for a highly dense microgel suspension (25.8% by weight). (b) Blow-up of the initial decay.
By determining a as a function of osmotic pressure, which corresponds to certain dextran concentration, we can estimate the particle bulk modulus [19]. We emphasize that this is only done in situation where Amgel >> A’slow, where we are certain that the slow decay is essentially dominated by the diffusion of the microgel particle. Using these microgels, we have recently started to explore the suspension dynamics at extremely high packings, which we can achieve due the ultrasoft character of these particles. In these experiments, we use cross-correlation in order to extract simple scattering from these multiply scattering samples. Interestingly, we find that despite the very high concentration used, these suspensions still are able to relax, as confirmed by the final relaxation of g1(t) shown in Fig. 4(a), which corresponds to a system at a concentration of 25.8% by weight. A closer look to the initial stages shows that there is also a faster relaxation, as shown in Fig. 4(b). By combining single-particle and suspension measurements, we hope to increase our current understanding of the behavior of dense microgel suspensions.
Acknowledgements
This work was supported by J.J. Lietor-Santos, B. Sierra-Martin and A. Fernandez-Nieves of the School of Physics, Georgia Institute of Technology.
References
[1] Tanaka T: Collapse of gels and critical end point, Physical Review Letters, 40, 820, 1978.
[2] Hirotsu S: Static and time-dependent properties of polymer gels around the volume phase transition, Phase Transitions, 47, 183, 1994.
[3] Saunders BR, Vincent B: Microgel particles as model colloids: Theory, properties and applications, Advances in Colloid and Interface Science, 80, 1, 1999.
[4] Nakamoto C, Kitada T, Kato E: Pressure dependence of the Flory-Huggins interaction parameter of poly(N-isopropylactrylamide) gels, Polymer gels and Networks, 4, 17, 1996.
[5] Budhlall BM, Marquez M, Velev OD: Microwave, Photo- and thermally responsive PNIPAm-Gold nanoparticle microgels, Langmuir, 24, 11959, 2008.
[6] Berne BJ, Pecora R: Dynamic light scattering, Dover, USA, 2000.
[7] Crocker JC, Grier DG: Methods of digital video microscopy for colloidal studies, Journal of Colloid and Interface Science, 179, 298, 1996.
[8] Senff H, Richtering W: Temperature sensitive microgel suspensions: Colloidal phase behaviour and rheology of soft spheres, Journal of Chemical Physics, 111, 1705, 1999.
[9] Das M, Zhang H, Kumacheva E: Microgels: Old materials with new applications, Annual Review of Material Research, 36, 117, 2006.
[10] Gottwald D, Likos CN, Kahl G, Lowen H: Phase behaviour of ionic microgels, Physical Review Letters, 92, 068301, 2004.
[11] Borrega R, Cloitre M, Betremieuz I, Ernst B, Leibler L: Concentration dependence of the low-shear viscosity of polyelectrolyte micro-networks: From hard spheres to soft microgels, Europhysics Letters, 47, 729, 1999.
[12] Likos CN: Effective interactions in soft condensed matter physics, Physics Reports- Review Section of Physics Letters, 348, 267, 2001.
[13] Schatzel K: Suppression of multiple scattering by photon cross-correlation techniques, Journal of Moder Optics, 38, 1849, 1991.
[14] Fernandez-Nieves A, Fernandez-Barbero, Vincent B, de las Nieves FJ: Charged controlled swelling of microgel particles, Macromolecules,33, 2114, 2000.
[15] In cross-correlation mode, the intercept of g2(t) – 1 is theoretically equal to 0.25 [16]. Misalignments in the experimental set-up and in the q for the two scattering experiments performed reduce this value typically to b » 0.2 . In these experiments, we obtain b » 0.17, indicating the presence of some multiple scattering.
[16] Claus Urban, Ph.D Thesis.
[17] Bonnet-Commet C, Belloni L, Cabane B: Osmotic-pressure of latex dispersions, Langmuir, 10, 4012, 1994.
[18] Ioan CE, Aberle T, Burchard W: Light scattering and viscosity behaviour of dextran in semidilute solution, Macromolecules, 34, 326, 2001.
[19] Hirotsu H: Softnening of bulk modulus and negative poisson ratio near the volume phase-transition of polymer gels, Journal of Chemical Physics, 94, 3949, 1991.
