LS Instruments | Introduction

Introduction

Rheology is the science that studies the behavior of fluids subject to a flow or a deformation. One of its main objectives is to comprehend how the microstructure of a fluid effects the response of that fluid to a deformation.

blood: a precious fluid with amazing rheological properties

Blood: a precious fluid with amazing rheological properties

Rheology is thus a discipline relevant to a wide range of fundamental processes in nature as well as many industrially relevant products including pharmaceutics, paints, inks, polymers, or cement. But it is also relevant to everyday consumer products such as food, cosmetics, house- and home-care products.

Gummi bears on why rheology matters to kids

Understanding the rheological properties of a fluid not only helps us to understand nature better but also facilitates the optimization of manufacturing processes and stimulates the discovery of novel intelligent materials that can often be used for many different applications.

Shampoo, you would not want it it be sticky

The rheological investigation of a fluid is typically conducted by applying a deformation to the fluid and analyzing the response to that deformation. Depending on the applied strain, the response of the system can be very different and conclusions can be drawn accordingly. This common approach is also referred to as mechanical or bulk rheology. It reveals the macrorheology of the fluid. Alternatively, the rheological properties can be measured by studying the Brownian motion of particles embedded in the system. This approach is called microrheology and is complementary to macrorheology [1, 2]. If the motion of the embedded particles is only caused by Browning motion, it is called passive microrheology. If an external force such as a magnetic or electric field is applied to displace the particles, one calls this active microrheology.

In the case of a macrorheological experiment, deformations can always be related to a combination of the two most simple deformations: shear and elongation.

Shear motion

The simplest case of a shear motion is represented in Figure 1a, where a fluid is confined between a fixed plate and a moving one.

In this case, the deformation $\gamma$ , is given by the ratio between the displacement of the material in the direction of the flow, s, and the distance between the two plates, h

$\gamma=\frac{s}{h}$

The rate of the deformation, named shear rate $\dot{\gamma}$ , is given by the ratio between the rate of the displacement v, and h:

$\dot{\gamma}=\frac{v}{h}$

Under shear motion, the shear stress, σ, and the first normal stress difference, $N_1$ , arise. The former acts in the same direction of the motion and it is proportional to the force $F$ , necessaire to move the plate as: $\sigma=\frac{F}{A}$ where A is the surface of the moving plate.

N₁is the result of two different normal stresses, one acting perpendicular to the shearing plane $\sigma_{n,1}$ , and the other acting perpendicular to the direction of the motion, $\sigma_{n,2}$ (Figure 1b). However, it is worth highlighting that normal stresses are only present in elastic fluids.

Figure 1. (a) Sketch of shear flow between parallel plates; (b) Shear normal stresses.

Elongational motion

When a fluid is subjected to an elongational motion, it undergoes stretching and compression. This is the case of all the materials going under extrusions processes, such as plastics, ceramics, pasta, etc.

Figure 2 shows a monoaxial elongational flow of a material which is held from one side and stretched from the other. This induces an elongation along the flow direction and a compression in the transversal ones.

As for the shear motion, it is possible to define the deformation, called Hencky strain, ε, calculated as: $\ln( \lambda )$ , where λis the elongational ratio (thus the final length L, on the initial one L₀), and the stretching rate as the time derivative of ε. The normal stress, σ, is defined as the ratio between the tractive force T, and the surface, S, to which it is applied as $\sigma=\frac{T}{S}$ .

The normal stress, in the elongational motion, can also be seen as the difference between the stress along the direction of the motion $\sigma_N$ , and that acting on the side surfaces, perpendicularly to the motion, $\sigma_L$

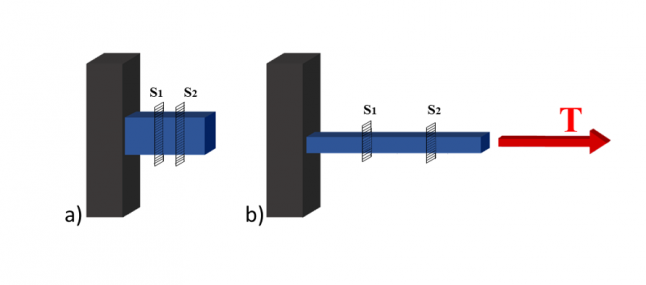
Figure 2. Extensional deformation.

[1] C.W. Macosko, Rheology: Principles, Measurements and Applications, VCH Publishers, New York 1994.
[2] Barnes H.A.; A Handbook of Elementary Rheology, The University of Wales Institute of Non-Newtonian Fluid Mechanics (2000).

Next Chapter